





Lost your password? Please enter your email address. You will receive a link and will create a new password via email.
Please briefly explain why you feel this question should be reported.
Please briefly explain why you feel this answer should be reported.
Please briefly explain why you feel this user should be reported.
Solve for in the inequality
in the inequality 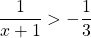
$\dfrac{1}{x+1}> -\dfrac{1}{3}$ cross multiply $1\times 3> -1(x+1)$ $\Rightarrow 3> -x-1$ collect like terms $x> -1-3$ $\Rightarrow x> -4$
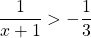
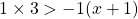
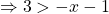
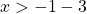
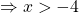
See lesscross multiply
collect like terms
Solve for in the inequality
in the inequality 
$7-3(x-1)< 13$ open up the bracket $7-3x+3< 13$ collect like terms $-3x< 13-7-3$ $\Rightarrow -3x \dfrac{3}{-3}$ $\therefore x> -1$

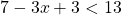
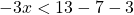
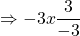
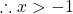
See lessopen up the bracket
collect like terms
Solve for in the inequality
in the inequality 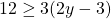
$12\geq 3(2y-3)$ open up the bracket $12\geq 6y-9$ add 9 to both sides $12+9\geq 6y$ $\Rightarrow 21\geq 6y$ divide both sides by 6 $\therefore \dfrac{21}{6}\geq y$ $y\leq \dfrac{7}{2}$
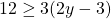
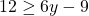
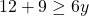
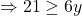
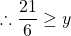
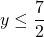
See lessopen up the bracket
add 9 to both sides
divide both sides by 6
Solve for in the inequality
in the inequality 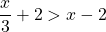
$\dfrac{x}{3}+2> x-2$ multiply through by the LCM (3) $3\times \dfrac{x}{3}+3\times 2> 3\times x-3\times2$ $\Rightarrow x+6> 3x-6$ collect like terms $x-3x>-6-6$ $\Rightarrow -2x> -12$ divide both side by -2 $\therefore x<6$
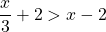
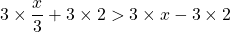
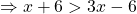
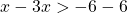
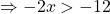
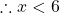
See lessmultiply through by the LCM (3)
collect like terms
divide both side by -2
Solve for in the inequality
in the inequality 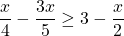
$\dfrac{x}{4}-\dfrac{3x}{5}\geq 3-\dfrac{x}{2}$ multiply through by the LCM (20) $20\times \dfrac{x}{4}-20\times \dfrac{3x}{5}\geq 20\times 3-20\times \dfrac{x}{2}$ $\Rightarrow 5x-12x\geq 60-10x$ collect like terms $5x-12x+10x\geq 60$ $\Rightarrow 3x\geq 60$ divide both side by 3 $\therefore x\geqRead more
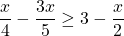
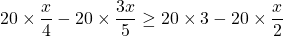
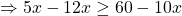
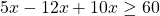
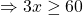

See lessmultiply through by the LCM (20)
collect like terms
divide both side by 3
Solve for in the inequality
in the inequality 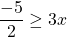
$\dfrac{-5}{2}\geq 3x$ cross multiply $-5\leq 2\times 3x$ $\Rightarrow -5\geq 6x$ divide both sides by 6 $\therefore \dfrac{-5}{6}\geq x$ $x\leq \dfrac{-5}{6}$
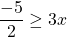
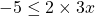
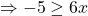
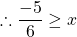
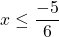
See lesscross multiply
divide both sides by 6
Solve for in the inequality
in the inequality 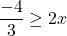
$\dfrac{-4}{3}\geq 2x$ cross multiply $-4\leq 3\times 2x$ $\Rightarrow -4\geq 6x$ divide both sides by 6 $\therefore \dfrac{-4}{6}\geq x$ $x\leq \dfrac{-2}{3}$
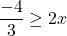
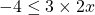
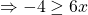
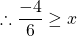
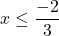
See lesscross multiply
divide both sides by 6
What is the value of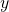 in
in 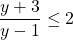 ?
?
$\dfrac{y+3}{y-1}\leq2$ cross multiply $y+3\leq 2(y-1)$ open up the bracket $y+3\leq 2y-2$ collect like terms $y-2y\leq -2-3$ $\Rightarrow -y\leq -5$ divide both sides by -1 $ \therefore y\geq 5$
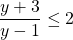
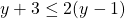
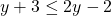
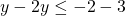
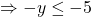
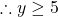
See lesscross multiply
open up the bracket
collect like terms
divide both sides by -1
Find the value of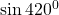
$\sin 420^{0}$ first step is to subtract 360 from the value above $\cos (420^{0}-360^{0})= \cos 60^{0}$ 60 falls in the first quadrant and sine is positive, using table $\therefore \sin 60^{0}=0.866$
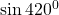
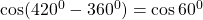
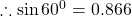
See lessfirst step is to subtract 360 from the value above
60 falls in the first quadrant and sine is positive,
using table
Find the value of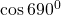
$\cos 690^{0}$ first step is to subtract 360 from the value above $\cos (690^{0}-360^{0})= \cos 330^{0}$ 330 falls in the fourth quadrant and cosine is positive $\Rightarrow +\cos (360^{0}-330^{0})=\cos 30^{0}$ using table $\therefore \cos 30^{0}=0.866$
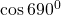
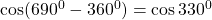
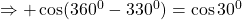
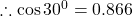
See lessfirst step is to subtract 360 from the value above
330 falls in the fourth quadrant and cosine is positive
using table