Home> Questions>Q 5912
Next
In Process

AcedStudy Latest Questions

Lwrnc
  • 0
Tyro
Asked: 2021-04-07T07:50:11+00:00 In:

Problem solving

  • 0

With the current, a boat travels 85 miles in 3 and half hours. Against the current, it travels 70 miles in 6 hours. Find the rate of the boat in still water.

question

You must login to add an answer.

Need An Account,

1 Answer

  2. acedstud
    This answer was edited.

    To solve this question, first recall the relationship between speed, distance, and time. That is,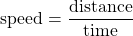 \text{speed}=\dfrac{\text{distance}}{\text{time}}.

    Next, we form the equations using the sentences we were given.

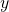    Let the speed of the boat in still water be x and the speed of the current be y, then since the boat travels 85 miles for 3 hours with the current, that is the combined speed (x + y) is 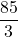\dfrac{85}{3} miles/hour. Also, since the boat travels 70 miles in 6 hours against the current, that is the difference in speed (x – y) is 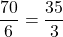\dfrac{70}{6}=\dfrac{35}{3} miles/hour..

    Thus, we have the equations:

    x+y=\dfrac{85}{3} . . . . . (1)

    x-y=\dfrac{35}{3} . . . . . (2)

    Adding the two equations gives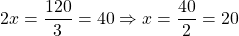 2x=\dfrac{120}{3}=40\Rightarrow x=\dfrac{40}{2}=20

    Therefore, the speed of the boat in still water is 20 miles/hour.

Related Questions