Find the derivative of the function ![]() .
.






Lost your password? Please enter your email address. You will receive a link and will create a new password via email.
Please briefly explain why you feel this question should be reported.
Please briefly explain why you feel this answer should be reported.
Please briefly explain why you feel this user should be reported.
You can use logarithmic differentiation
Take the natural logarithm of both sides
ln y=ln xx
properties of logarithms, rewrite the right hand side
ln y=x ln x
Differentiate both sides with respect to x
Use the on the right side
1/y dy/dx=ln x+x (1/x)
1/y dy/dx=ln x+1
Multiply both sides by y
dy/dx=y(ln x+1)
Now y=xx so we can write
dy/dx=xx(ln x+1)
To find the derivative of the function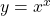 , first, we use the logarithm property to evaluate the exponent.
, first, we use the logarithm property to evaluate the exponent.
Thus, taking the logarithm of both sides, we have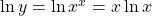
Evaluating the derivative of both sides gives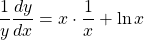 [*Note than the right-hand side is a product so we used the product rule of differentiation.]
[*Note than the right-hand side is a product so we used the product rule of differentiation.]
Solving algebraically, we have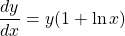 .
.
But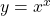 , so we have
, so we have 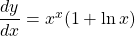 .
.
Therefore, the derivative of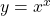 is
is 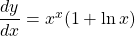 .
.